15 - Conduction - Fins

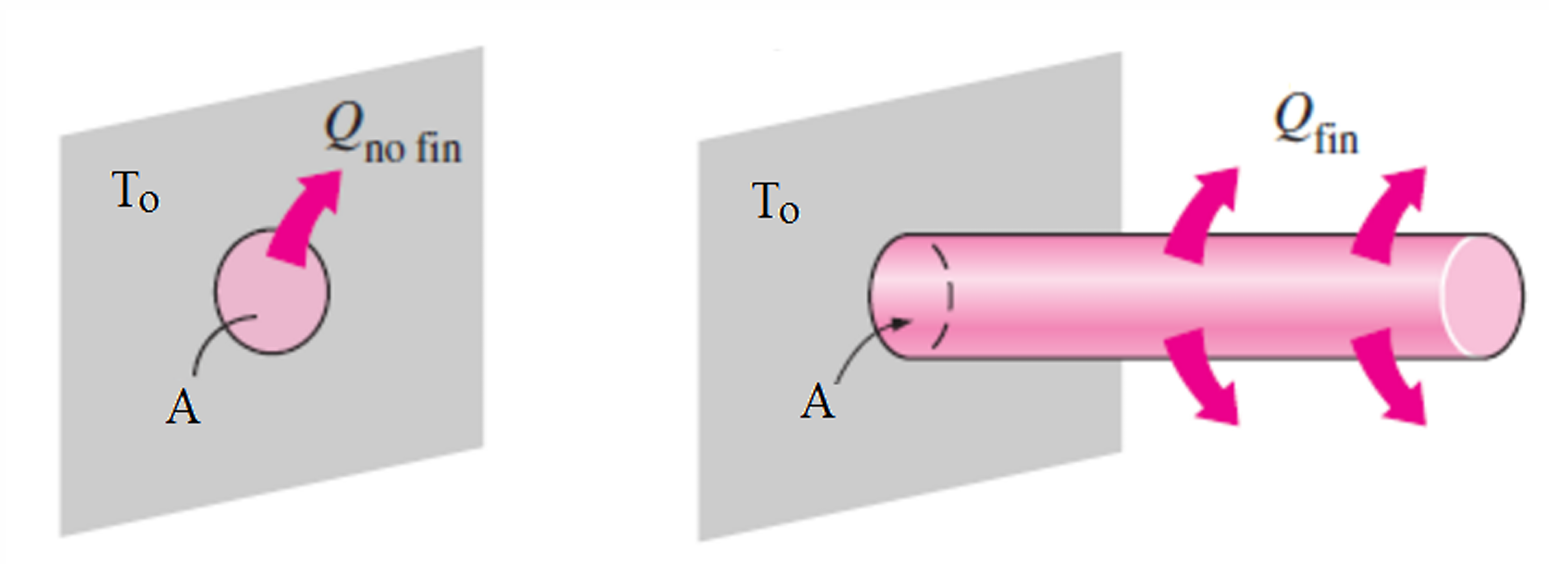
The performance of fins is judged on the basis of the enhancement in heat transfer relative to the no-fin case, and expressed in terms of the fin effectiveness:

\[\varepsilon_{\text{fin}} = \frac{Q_{\text{fin}}}{Q_{\text{no fin}}} = \frac{\text{heat transfer rate with fin}}{\text{heat transfer rate without fin}}\]
\[\varepsilon_{\text{fin}} = \left\{ \begin{array}{ll}<1 & \text{fin act as insulation} \\ = 1 & \text{fin does not affect heat transfer} \\ >1 & \text{fin enhances heat transfer} \end{array} \right.\]

\[ {\eta_{\text{fin}} < 1 \qquad \text{ but } \qquad \varepsilon_{\text{fin}} > 1} \]

For the long-fin \[\varepsilon_{\text{fin}} = \frac{Q_{\text{fin}}}{Q_{\text{no fin}}} = \frac{\sqrt{PhkA}(T_o-T_\infty)}{hA(T_o-T_\infty)} = \frac{\sqrt{PhkA}}{hA} = \sqrt{\frac{kP}{hA}}\] and, \[\frac{\varepsilon_{\text{fin}}}{\eta_{\text{fin}}} = \frac{PL}{A}\]

Effectiveness of fin must be greater than 2; otherwise don’t use the fin.