18 - Conduction - Unsteady Heat Conduction
Completion requirements
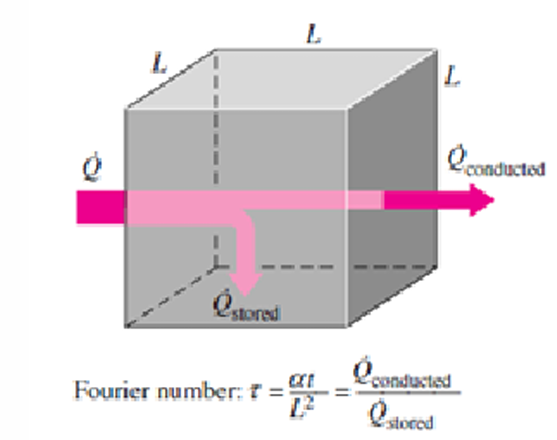
6. Fourier Number (Fo)
\[\tau = \text{Fo} = \frac{\alpha t}{L^2} = \frac{k(1/L)L^2\; \Delta T}{\rho C_PL^3 \; \Delta T/t} = \frac{\begin{array}{c}\text{rate of heat conduction}\\ \text{across $L$ in volume $L^3$} \end{array}}{\begin{array}{c}\text{rate of heat storage}\\ \text{in volume $L^3$} \end{array}}\] Fourier number is a measure of heat conducted through a body relative to heat stored. Thus, a large value of the Fourier number indicates faster propagation of heat through a body.