32 - Boiling
2. Pool Boiling
Pool boiling is a process in which the heating surface is submerged in a large body of stagnant liquid. The relative motion of the vapor produced and the surrounding liquid near the heating surface is due primarily to the buoyancy effect of the vapor. Nevertheless, the body of the liquid as a whole is essentially at rest.
By force balance, it can be shown that the vapor pressure within the bubble must be somewhat higher than the pressure of the surrounding liquid. It follows that the vapor (and liquid) temperature must be somewhat higher than the saturation temperature, \(T_{\text{sat}}\), corresponding to the liquid (or system) pressure.
Using the Clausius-Clapeyron equation to relate saturation temperatures and pressures, it can then be shown that the magnitude of the superheat \((T_{\text{nucleation}}-T_{\text{sat}})\) required to sustain the bubble is inversely proportional to the radius of the bubbles, \(r_{\text{bubble}}\): \[T_{\text{nucleation}}-T_{\text{sat}} \propto \frac{1}{r_{\text{bubble}}}\] This shows that the smaller the bubble, the higher is the superheat required for nucleation
The boiling process consists of the following steps:
-
Nucleation
-
Bubble growth
-
Bubble departure from the surface
-
Collapse or further growth depending upon whether the bubble liquid is sub-cooled or super heated
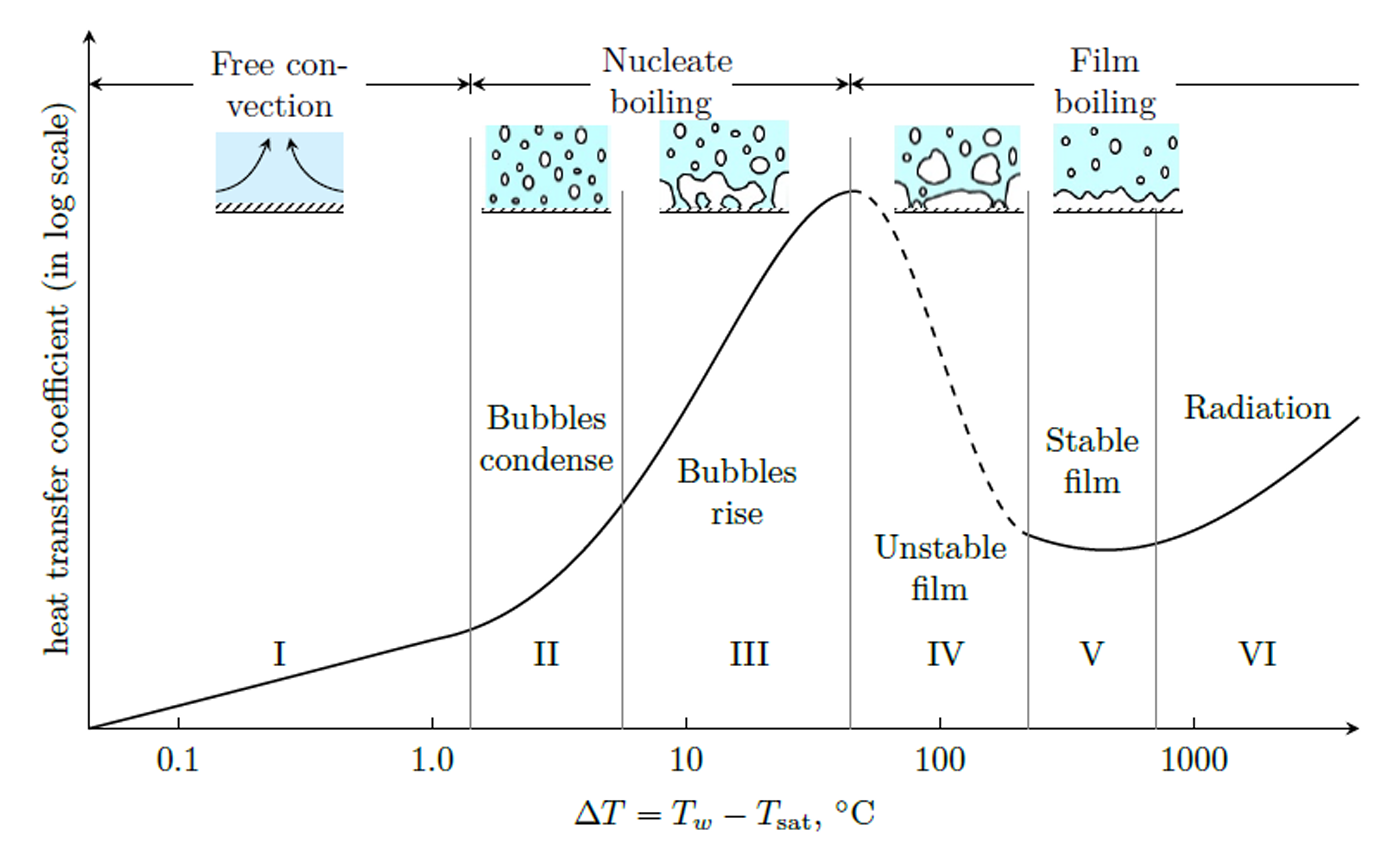
Typical boiling curve for water at 1 atm. Note: the \(x\)-scale is logarithmic!
