Instant Notes: 7. Evaporation
7.1 Mass and Heat Balances for a Evaporator
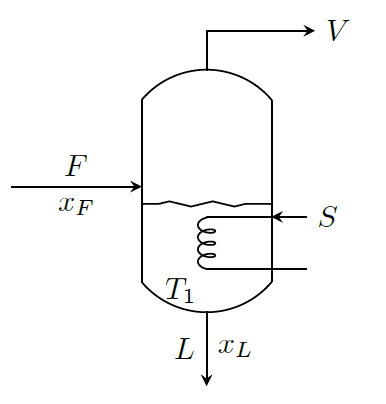
Material Balance: \[\begin{aligned} & \text{Overall balance:} \qquad & F &= L + V \\ & \text{Balance on solute:} & F\;x_F &= L\;x_L\end{aligned}\] where \(x\) is the concentration of solute.
Energy Balance: \[\begin{aligned} FH_F + SH_S &= LH_L + VH_V + SH_C \\ FH_F + S(H_S-H_C) &= LH_L + VH_V \\ FH_F + S\lambda_S &= LH_L + VH_V \end{aligned}\] By taking reference temperature as that of boiling point of solution, we get \[ \begin{aligned} FC_P(T_F-T_1) + S\lambda_S &= L\times0 + V\lambda_V \\ \Longrightarrow \quad S\lambda_S &= V\lambda_V + FC_P(T_1-T_F)\end{aligned}\] where \(\lambda_V\) is the latent heat of vaporization of water vapor at \(T_1\).
If feed is at \(T_1\) (i.e., at the boiling point of solution), then, \[S\lambda_S = V\lambda_V\]
