Rate Equation from CSTR data

From the following data find a satisfactory rate equation for the gas-phase decomposition of pure A, A -> R + S, in a mixed flow reactor.

Calculations:

For the mixed flow reactor, the design equation is,

τ = C_AoX_A/-r_A → 1

For variable density systems,

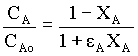
Where, ε_A = fractional change in volume = (V_{XA = 1} - V_{XA = 0}) / V_{XA = 0} = (2 - 1)/1 = 1

Therefore,

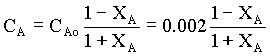 à 2

For the first order equation, equn.1 can be written as

τ = C_AoX_A/ kC_A → 3

substituting for C_Ao in equation 3,

τ = 0.002 X_A/ kC_A

substituting for C_A from equn.2,

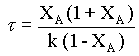
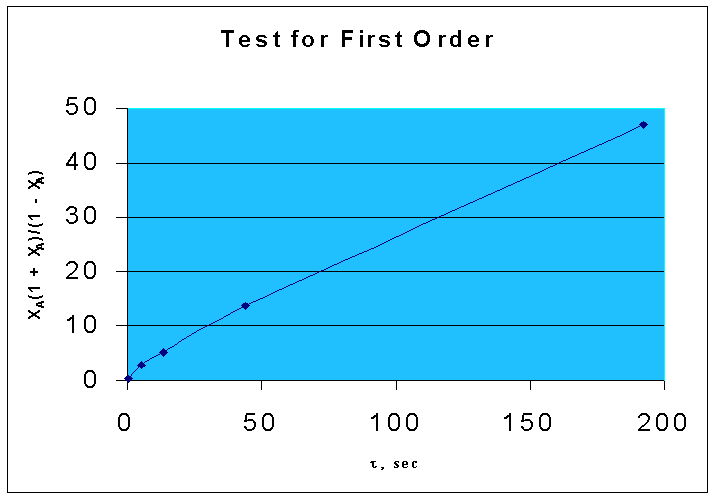
That is, if the given data is for a first order reaction, τ vs. X_A(1 + X_A)/(1 - X_A) will be a straight line, with a slope of k.

From the above data, the following graph is drawn :(graph 1)

For the second order equation, equn.1 can be written as

τ = C_AoX_A/ kC_A²

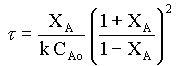
That is, if the given data is for a second order reaction, τ vs. X_A(1 + X_A)²/ (1 - X_A)² will be a straight line with a slope of kC_Ao.

From the above data, the following graph is drawn :(graph 2)

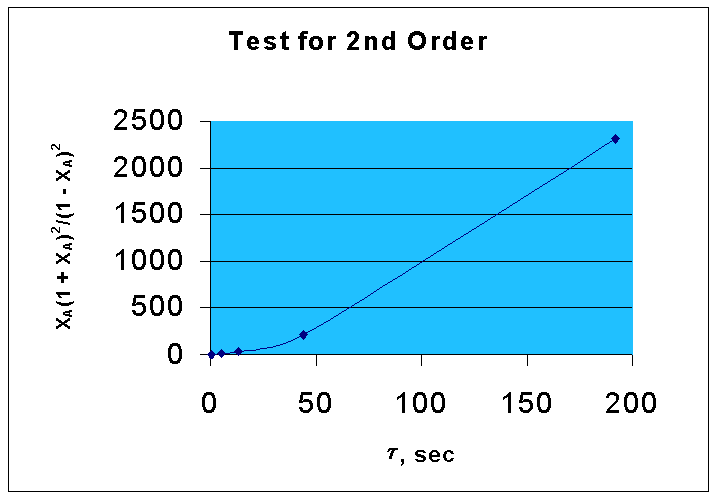
By comparing two graphs it is seen that first order kinetics is well fitting the given data.

That is, the reaction is following first order.

Slope = 0.2493 (from the graph.1)

Therefore,

k = 0.2493 sec^-1.

Therefore, rate equation for the given reaction is

-r_A = 0.2493 C_Ao (1 - X_A)/(1 + X_A) = 4.986 x 10^-4 (1 - X_A)/(1 + X_A) mol/lit.sec