Euler's Equation of Motion
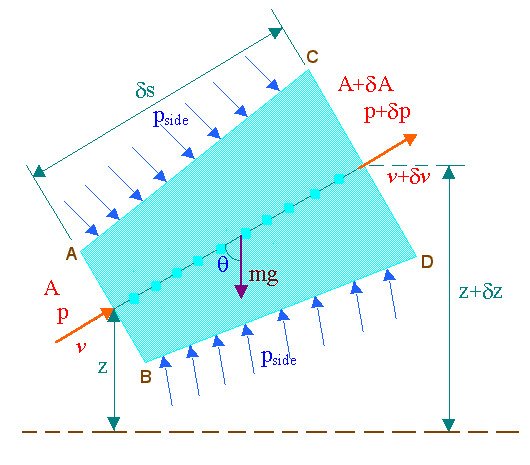
Mass in per unit time = \(\rho\)Av = \(\dot m\)
For steady flow, mass out per unit time = \(\dot m\)
Rate of momentum in = \(\dot m\)v
Rate of momentum out = \[\dot m (v + \delta v)\]
Rate of increase of momentum from AB to CD = \[\dot m (v + \delta v)\] \(\dot m\)v = \(\rho\)Av \(\delta\)v → 1
Force due to p in the direction of motion = pA
Force due to p + \(\delta\)p opposing the direction of motion = (p + \(\delta\)p)(A + \(\delta\)A)
Force due to pside producing a component in the direction of motion = pside\(\delta\)A
Force due to mg producing a component opposing the direction of motion = mg\(\cos\)(\(\theta\))
Resultant force in the direction of motion = pA - (p + \(\delta\)p)(A + \(\delta\)A) + pside\(\delta\)A - mg\(\cos\)(\(\theta\)) → 2
The value of pside will vary from p at AB to p + \(\delta\)p at CD, and can be taken as p + k\(\delta\)p where k is fraction.
Mass of fluid element \[ABCD = m = \rho g(A + \frac{1}{2} \delta A) \delta s\]
And \[\delta s = \frac{\delta z}{\cos(\theta)}; since \cos(\theta) = \frac{\delta z}{\delta s}\]
Substituting in equn.2,
Resultant force in the direction of motion = pA - (p + \(\delta\)) (A + \(\delta\) A) + p + k \(\delta\) p - \(\rho\) g(A + \[\frac{1}{2}\] \(\delta\) A) \(\delta\) z
= -A\(\delta\) p - \(\delta\) p \(\delta\) A + k \(\delta\) p \(\delta\) A - \(\rho\) gA\(\delta\) z - \[\frac{1}{2}\] \(\delta\) A \(\delta\) z
Neglecting products of small quantities,
Resultant force in the direction of motion = -A\(\delta\)p - \(\rho\)gA\(\delta\)z → 3
Applying Newton's second law, (i.e., equating equns.1 & 3)
\(\rho\)Av dv = -A\(\delta\)p - \(\rho\)gA\(\delta\)z
dividing by \(\rho\)A\(\delta\)s,
\[\frac{v \delta \delta}{\delta s} = - \frac{1}{\rho} \frac{\delta p}{\delta s} - g \frac{\delta z}{\delta s}\]
\[\frac{vdv}{ds} + \frac{1}{\rho}\frac{dp}{ds} + g \frac{dz}{ds} = 0\]
or, in the limit as ds → 0,
\[\frac{1}{\rho} \frac{dp}{ds} + \frac{vdv}{ds} + g \frac{dz}{ds} = 0\]
This is known as Euler's equation, giving, in differential form
\[\frac{dp}{\rho} + vdv + gdz = 0\]
the relationship between p, v, r and elevation z, along a streamline for steady flow.
It can not be integrated until the relationship density and pressure is known.
For incompressible fluid, r is constant; therefore the Euler's equation is integrated to give the following:
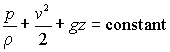
\[\frac{p}{\rho} + \frac{v^2}{2} + gz = constant\]
which is nothing but the Bernoulli equation.
